Math Mindsets: Encouraging Curious & Creative Problem-Solving

Engage students in rich mathematical tasks that honor the process more than the solution. 

VICTORIA FERNANDEZ
Lead Professional Development Coach

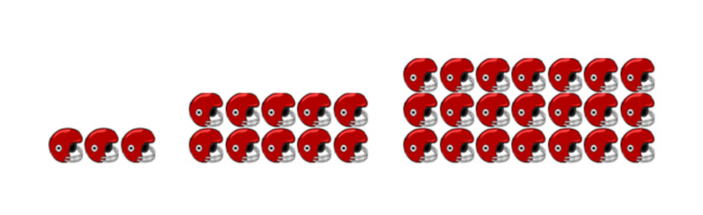
​I was recently sitting with a high school algebra teacher — who was preparing a lesson about translating sequences into functions or equations — and trying to encourage him to use this archive of visual patterns with his students. We discussed matching different groups of students to different images and asking them to try writing an equation for the pattern. As the teacher started practicing on his own, writing equations for some of the patterns that were offered, this picture came up, and we both began to struggle. 

​Neither of us could figure it out.

Other teachers started coming by to join:

“There has to be an exponent in there somewhere...”
“But just an exponent wouldn’t make sense…”
“Are we multiplying the exponent? Or maybe multiplying and adding?”

This had started with only one teacher, but soon there were five teachers huddled around this image, trying to find a solution. One teacher got out a marker and began writing possibilities on the board. Another started running through calculations in their head, shouting out theories. Finally, one teacher said, “Oh, it’s a quadratic!” 

The algebra teacher who had started with me stated, “see I can’t do this with my students, we couldn’t even figure it out”. But I had the complete opposite reaction. I was surprised at how many had engaged in our puzzle. We were all trying out different methods, debating and discussing throughout our process.

​This is what a math classroom should look like every day. 

---

Making room for authentic engagement

As math teachers, it can feel scary to introduce a problem to students that doesn’t have a clear and simple solution. But how else is authentic math engagement supposed to happen in the classroom? In our example, even if we had never arrived at a solution, all the math thinking and practice we did to try and get there would have been worth the struggle. Even if a student never achieved a correct equation or solution, they still would have stretched their thinking and understanding of patterns and functions just by trying to work through the task.

The inspiration for this task came from Jo Boaler’s work on Mathematical Mindsets. In her book, she reminds us how beautiful and rich mathematical thinking can be, and offers advice on how to bring that into your classroom. This task we were testing out among teachers includes many of the suggestions she offers for making rich mathematical tasks. Boaler describes these tasks as having multiple entry points, visual components, and options for inquiry and debate. 

As you incorporate these elements into the math tasks in your classroom, consider the following questions as you shift into a richer mathematical lens:

• Is there only one way to approach this task? Can I change this task to have multiple solutions or multiple processes for finding the solution? 
• Is there a visual component in this assignment? Can I add a visual representation or graph to help students work through the task? 
• Is there room for debate and discussion in this assignment? Could I add a place where students have to defend their mathematical thinking to a critic? 
• Do I show students how to solve the problem before presenting it? Can I rearrange my lesson to allow students to play with a problem before offering them processes and solutions? 

Our original task starts with a visual component and includes multiple methods of entry. Students have the opportunity to make sense of the visual in any way they see fit — maybe they prefer to focus on the numbers, or maybe they want to look at the way the shape of the image is changing. There are no clear steps to follow to solve this puzzle; students have to play and engage in multiple ways before they can predict patterns and solutions. 

Another great aspect of this task is that students of all math levels can participate and still walk away learning something from the experience. Maybe some students will leave noticing there is not a constant amount of change between the pictures. Maybe some students will consider the way addition and multiplication look different in patterns. Maybe some students will write a quadratic equation and then be able to predict future images. The possibilities are endless. 

---

When we provide math problems that have very clear and explicit steps, we may lead students to the correct answer, but we also limit the creativity they can experience. We teach students that there is a right and wrong way to do math. We contribute to the negative feelings and attitudes many students already have about math. And lastly, we take away the fun. 

Making room for rich mathematical tasks that have multiple entry points, opportunities for debate, and visual components can help make every student feel like they can one day be a real mathematician. It can help students of all levels rediscover the fun in math.

MAXIMIZING MATH TALK

DISCUSSING LITERACY IN MATH

ENGAGING ACROSS CONTENT AREAS